普通最小二乘法¶
[1]:
%matplotlib inline
[2]:
import matplotlib.pyplot as plt
import numpy as np
import pandas as pd
import statsmodels.api as sm
np.random.seed(9876789)
OLS 估计¶
人工数据
[3]:
nsample = 100
x = np.linspace(0, 10, 100)
X = np.column_stack((x, x ** 2))
beta = np.array([1, 0.1, 10])
e = np.random.normal(size=nsample)
我们的模型需要截距,所以我们添加了一列 1
[4]:
X = sm.add_constant(X)
y = np.dot(X, beta) + e
拟合和摘要
[5]:
model = sm.OLS(y, X)
results = model.fit()
print(results.summary())
OLS Regression Results
==============================================================================
Dep. Variable: y R-squared: 1.000
Model: OLS Adj. R-squared: 1.000
Method: Least Squares F-statistic: 4.020e+06
Date: Thu, 03 Oct 2024 Prob (F-statistic): 2.83e-239
Time: 15:44:50 Log-Likelihood: -146.51
No. Observations: 100 AIC: 299.0
Df Residuals: 97 BIC: 306.8
Df Model: 2
Covariance Type: nonrobust
==============================================================================
coef std err t P>|t| [0.025 0.975]
------------------------------------------------------------------------------
const 1.3423 0.313 4.292 0.000 0.722 1.963
x1 -0.0402 0.145 -0.278 0.781 -0.327 0.247
x2 10.0103 0.014 715.745 0.000 9.982 10.038
==============================================================================
Omnibus: 2.042 Durbin-Watson: 2.274
Prob(Omnibus): 0.360 Jarque-Bera (JB): 1.875
Skew: 0.234 Prob(JB): 0.392
Kurtosis: 2.519 Cond. No. 144.
==============================================================================
Notes:
[1] Standard Errors assume that the covariance matrix of the errors is correctly specified.
您可以直接从拟合模型中提取感兴趣的量。键入 dir(results) 以获取完整列表。以下是一些示例
[6]:
print("Parameters: ", results.params)
print("R2: ", results.rsquared)
Parameters: [ 1.34233516 -0.04024948 10.01025357]
R2: 0.9999879365025871
OLS 非线性曲线但参数线性¶
我们模拟了 x 和 y 之间具有非线性关系的人工数据
[7]:
nsample = 50
sig = 0.5
x = np.linspace(0, 20, nsample)
X = np.column_stack((x, np.sin(x), (x - 5) ** 2, np.ones(nsample)))
beta = [0.5, 0.5, -0.02, 5.0]
y_true = np.dot(X, beta)
y = y_true + sig * np.random.normal(size=nsample)
拟合和摘要
[8]:
res = sm.OLS(y, X).fit()
print(res.summary())
OLS Regression Results
==============================================================================
Dep. Variable: y R-squared: 0.933
Model: OLS Adj. R-squared: 0.928
Method: Least Squares F-statistic: 211.8
Date: Thu, 03 Oct 2024 Prob (F-statistic): 6.30e-27
Time: 15:44:50 Log-Likelihood: -34.438
No. Observations: 50 AIC: 76.88
Df Residuals: 46 BIC: 84.52
Df Model: 3
Covariance Type: nonrobust
==============================================================================
coef std err t P>|t| [0.025 0.975]
------------------------------------------------------------------------------
x1 0.4687 0.026 17.751 0.000 0.416 0.522
x2 0.4836 0.104 4.659 0.000 0.275 0.693
x3 -0.0174 0.002 -7.507 0.000 -0.022 -0.013
const 5.2058 0.171 30.405 0.000 4.861 5.550
==============================================================================
Omnibus: 0.655 Durbin-Watson: 2.896
Prob(Omnibus): 0.721 Jarque-Bera (JB): 0.360
Skew: 0.207 Prob(JB): 0.835
Kurtosis: 3.026 Cond. No. 221.
==============================================================================
Notes:
[1] Standard Errors assume that the covariance matrix of the errors is correctly specified.
提取其他感兴趣的量
[9]:
print("Parameters: ", res.params)
print("Standard errors: ", res.bse)
print("Predicted values: ", res.predict())
Parameters: [ 0.46872448 0.48360119 -0.01740479 5.20584496]
Standard errors: [0.02640602 0.10380518 0.00231847 0.17121765]
Predicted values: [ 4.77072516 5.22213464 5.63620761 5.98658823 6.25643234 6.44117491
6.54928009 6.60085051 6.62432454 6.6518039 6.71377946 6.83412169
7.02615877 7.29048685 7.61487206 7.97626054 8.34456611 8.68761335
8.97642389 9.18997755 9.31866582 9.36587056 9.34740836 9.28893189
9.22171529 9.17751587 9.1833565 9.25708583 9.40444579 9.61812821
9.87897556 10.15912843 10.42660281 10.65054491 10.8063004 10.87946503
10.86825119 10.78378163 10.64826203 10.49133265 10.34519853 10.23933827
10.19566084 10.22490593 10.32487947 10.48081414 10.66779556 10.85485568
11.01006072 11.10575781]
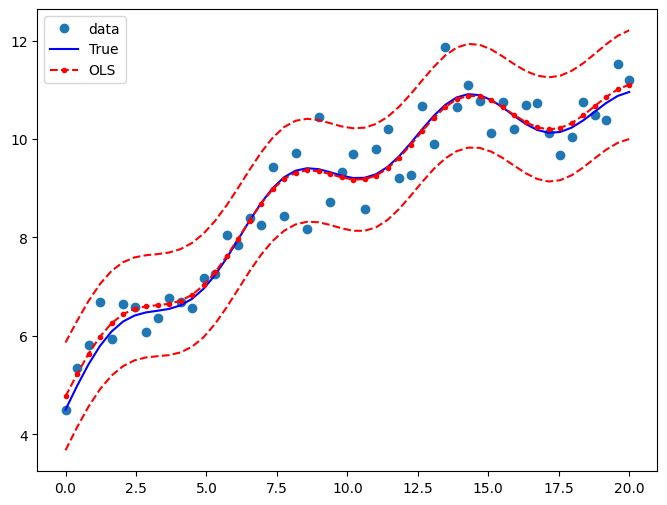
绘制图表以将真实关系与 OLS 预测进行比较。预测周围的置信区间是使用 wls_prediction_std 命令构建的。
[10]:
pred_ols = res.get_prediction()
iv_l = pred_ols.summary_frame()["obs_ci_lower"]
iv_u = pred_ols.summary_frame()["obs_ci_upper"]
fig, ax = plt.subplots(figsize=(8, 6))
ax.plot(x, y, "o", label="data")
ax.plot(x, y_true, "b-", label="True")
ax.plot(x, res.fittedvalues, "r--.", label="OLS")
ax.plot(x, iv_u, "r--")
ax.plot(x, iv_l, "r--")
ax.legend(loc="best")
[10]:
<matplotlib.legend.Legend at 0x7fe3f3907c70>
带虚拟变量的 OLS¶
我们生成一些人工数据。有 3 组将使用虚拟变量进行建模。第 0 组是省略/基准类别。
[11]:
nsample = 50
groups = np.zeros(nsample, int)
groups[20:40] = 1
groups[40:] = 2
# dummy = (groups[:,None] == np.unique(groups)).astype(float)
dummy = pd.get_dummies(groups).values
x = np.linspace(0, 20, nsample)
# drop reference category
X = np.column_stack((x, dummy[:, 1:]))
X = sm.add_constant(X, prepend=False)
beta = [1.0, 3, -3, 10]
y_true = np.dot(X, beta)
e = np.random.normal(size=nsample)
y = y_true + e
检查数据
[12]:
print(X[:5, :])
print(y[:5])
print(groups)
print(dummy[:5, :])
[[0. 0. 0. 1. ]
[0.40816327 0. 0. 1. ]
[0.81632653 0. 0. 1. ]
[1.2244898 0. 0. 1. ]
[1.63265306 0. 0. 1. ]]
[ 9.28223335 10.50481865 11.84389206 10.38508408 12.37941998]
[0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1
1 1 1 2 2 2 2 2 2 2 2 2 2]
[[ True False False]
[ True False False]
[ True False False]
[ True False False]
[ True False False]]
拟合和摘要
[13]:
res2 = sm.OLS(y, X).fit()
print(res2.summary())
OLS Regression Results
==============================================================================
Dep. Variable: y R-squared: 0.978
Model: OLS Adj. R-squared: 0.976
Method: Least Squares F-statistic: 671.7
Date: Thu, 03 Oct 2024 Prob (F-statistic): 5.69e-38
Time: 15:44:51 Log-Likelihood: -64.643
No. Observations: 50 AIC: 137.3
Df Residuals: 46 BIC: 144.9
Df Model: 3
Covariance Type: nonrobust
==============================================================================
coef std err t P>|t| [0.025 0.975]
------------------------------------------------------------------------------
x1 0.9999 0.060 16.689 0.000 0.879 1.121
x2 2.8909 0.569 5.081 0.000 1.746 4.036
x3 -3.2232 0.927 -3.477 0.001 -5.089 -1.357
const 10.1031 0.310 32.573 0.000 9.479 10.727
==============================================================================
Omnibus: 2.831 Durbin-Watson: 1.998
Prob(Omnibus): 0.243 Jarque-Bera (JB): 1.927
Skew: -0.279 Prob(JB): 0.382
Kurtosis: 2.217 Cond. No. 96.3
==============================================================================
Notes:
[1] Standard Errors assume that the covariance matrix of the errors is correctly specified.
绘制图表以将真实关系与 OLS 预测进行比较
[14]:
pred_ols2 = res2.get_prediction()
iv_l = pred_ols2.summary_frame()["obs_ci_lower"]
iv_u = pred_ols2.summary_frame()["obs_ci_upper"]
fig, ax = plt.subplots(figsize=(8, 6))
ax.plot(x, y, "o", label="Data")
ax.plot(x, y_true, "b-", label="True")
ax.plot(x, res2.fittedvalues, "r--.", label="Predicted")
ax.plot(x, iv_u, "r--")
ax.plot(x, iv_l, "r--")
legend = ax.legend(loc="best")

联合假设检验¶
F 检验¶
我们要检验虚拟变量的系数都等于零的假设,即 \(R \times \beta = 0\)。F 检验使我们强烈拒绝 3 组中常数相同的零假设
[15]:
R = [[0, 1, 0, 0], [0, 0, 1, 0]]
print(np.array(R))
print(res2.f_test(R))
[[0 1 0 0]
[0 0 1 0]]
<F test: F=145.49268198027963, p=1.2834419617282974e-20, df_denom=46, df_num=2>
您还可以使用类似公式的语法来检验假设
[16]:
print(res2.f_test("x2 = x3 = 0"))
<F test: F=145.49268198027949, p=1.2834419617283214e-20, df_denom=46, df_num=2>
小组效应小¶
如果我们生成具有较小组效应的人工数据,T 检验将无法再拒绝零假设
[17]:
beta = [1.0, 0.3, -0.0, 10]
y_true = np.dot(X, beta)
y = y_true + np.random.normal(size=nsample)
res3 = sm.OLS(y, X).fit()
[18]:
print(res3.f_test(R))
<F test: F=1.224911192540883, p=0.30318644106312964, df_denom=46, df_num=2>
[19]:
print(res3.f_test("x2 = x3 = 0"))
<F test: F=1.2249111925408838, p=0.30318644106312964, df_denom=46, df_num=2>
多重共线性¶
Longley 数据集以高度的多重共线性而闻名。也就是说,外生预测变量高度相关。这是有问题的,因为它会影响我们的系数估计的稳定性,因为我们对模型规范进行了微小的更改。
[20]:
from statsmodels.datasets.longley import load_pandas
y = load_pandas().endog
X = load_pandas().exog
X = sm.add_constant(X)
拟合和摘要
[21]:
ols_model = sm.OLS(y, X)
ols_results = ols_model.fit()
print(ols_results.summary())
OLS Regression Results
==============================================================================
Dep. Variable: TOTEMP R-squared: 0.995
Model: OLS Adj. R-squared: 0.992
Method: Least Squares F-statistic: 330.3
Date: Thu, 03 Oct 2024 Prob (F-statistic): 4.98e-10
Time: 15:44:51 Log-Likelihood: -109.62
No. Observations: 16 AIC: 233.2
Df Residuals: 9 BIC: 238.6
Df Model: 6
Covariance Type: nonrobust
==============================================================================
coef std err t P>|t| [0.025 0.975]
------------------------------------------------------------------------------
const -3.482e+06 8.9e+05 -3.911 0.004 -5.5e+06 -1.47e+06
GNPDEFL 15.0619 84.915 0.177 0.863 -177.029 207.153
GNP -0.0358 0.033 -1.070 0.313 -0.112 0.040
UNEMP -2.0202 0.488 -4.136 0.003 -3.125 -0.915
ARMED -1.0332 0.214 -4.822 0.001 -1.518 -0.549
POP -0.0511 0.226 -0.226 0.826 -0.563 0.460
YEAR 1829.1515 455.478 4.016 0.003 798.788 2859.515
==============================================================================
Omnibus: 0.749 Durbin-Watson: 2.559
Prob(Omnibus): 0.688 Jarque-Bera (JB): 0.684
Skew: 0.420 Prob(JB): 0.710
Kurtosis: 2.434 Cond. No. 4.86e+09
==============================================================================
Notes:
[1] Standard Errors assume that the covariance matrix of the errors is correctly specified.
[2] The condition number is large, 4.86e+09. This might indicate that there are
strong multicollinearity or other numerical problems.
/opt/hostedtoolcache/Python/3.10.15/x64/lib/python3.10/site-packages/scipy/stats/_axis_nan_policy.py:418: UserWarning: `kurtosistest` p-value may be inaccurate with fewer than 20 observations; only n=16 observations were given.
return hypotest_fun_in(*args, **kwds)
条件数¶
评估多重共线性的一种方法是计算条件数。大于 20 的值令人担忧(参见 Greene 4.9)。第一步是将自变量归一化为具有单位长度
[22]:
norm_x = X.values
for i, name in enumerate(X):
if name == "const":
continue
norm_x[:, i] = X[name] / np.linalg.norm(X[name])
norm_xtx = np.dot(norm_x.T, norm_x)
然后,我们取最大特征值与最小特征值之比的平方根。
[23]:
eigs = np.linalg.eigvals(norm_xtx)
condition_number = np.sqrt(eigs.max() / eigs.min())
print(condition_number)
56240.87037739987
删除观测值¶
Greene 还指出,删除单个观测值会对系数估计产生重大影响
[24]:
ols_results2 = sm.OLS(y.iloc[:14], X.iloc[:14]).fit()
print(
"Percentage change %4.2f%%\n"
* 7
% tuple(
[
i
for i in (ols_results2.params - ols_results.params)
/ ols_results.params
* 100
]
)
)
Percentage change 4.55%
Percentage change -105.20%
Percentage change -3.43%
Percentage change 2.92%
Percentage change 3.32%
Percentage change 97.06%
Percentage change 4.64%
我们也可以查看为此类统计数据的正式统计数据,例如 DFBETAS - 当删除该观测值时,每个系数变化量的标准化度量。
[25]:
infl = ols_results.get_influence()
通常,我们可能认为绝对值大于 \(2/\sqrt{N}\) 的 DBETAS 是有影响力的观测值
[26]:
2.0 / len(X) ** 0.5
[26]:
0.5
[27]:
print(infl.summary_frame().filter(regex="dfb"))
dfb_const dfb_GNPDEFL dfb_GNP dfb_UNEMP dfb_ARMED dfb_POP dfb_YEAR
0 -0.016406 -0.234566 -0.045095 -0.121513 -0.149026 0.211057 0.013388
1 -0.020608 -0.289091 0.124453 0.156964 0.287700 -0.161890 0.025958
2 -0.008382 0.007161 -0.016799 0.009575 0.002227 0.014871 0.008103
3 0.018093 0.907968 -0.500022 -0.495996 0.089996 0.711142 -0.040056
4 1.871260 -0.219351 1.611418 1.561520 1.169337 -1.081513 -1.864186
5 -0.321373 -0.077045 -0.198129 -0.192961 -0.430626 0.079916 0.323275
6 0.315945 -0.241983 0.438146 0.471797 -0.019546 -0.448515 -0.307517
7 0.015816 -0.002742 0.018591 0.005064 -0.031320 -0.015823 -0.015583
8 -0.004019 -0.045687 0.023708 0.018125 0.013683 -0.034770 0.005116
9 -1.018242 -0.282131 -0.412621 -0.663904 -0.715020 -0.229501 1.035723
10 0.030947 -0.024781 0.029480 0.035361 0.034508 -0.014194 -0.030805
11 0.005987 -0.079727 0.030276 -0.008883 -0.006854 -0.010693 -0.005323
12 -0.135883 0.092325 -0.253027 -0.211465 0.094720 0.331351 0.129120
13 0.032736 -0.024249 0.017510 0.033242 0.090655 0.007634 -0.033114
14 0.305868 0.148070 0.001428 0.169314 0.253431 0.342982 -0.318031
15 -0.538323 0.432004 -0.261262 -0.143444 -0.360890 -0.467296 0.552421
上次更新:2024 年 10 月 3 日